New publication in Nature Physics with JINR participation
News, 30 April 2020
On 13 April, a scientific paper by an international scientific team was published in Nature Physics. A LIT staff member O. Chuluunbaatar and a BLTP employee Yu. V. Popov were members of the group in the frames of JINR international cooperation. The group conducted a kinematically complete experimental measurement of characteristics of Compton scattering at free atoms using the highly efficient method of COLD Target Recoil Ion Momentum Spectroscopy (COLTRIMS). The group also provided a relevant theoretical description of it.
Experimentalists from the Goethe University (Frankfurt am Main, Germany) targeted a strong photon beam of the Petra III synchrotron (DESY, Hamburg) through the helium supersonic flow.
The COLTRIMS method allowed measuring not only the scattered electron momentum but also the momentum of helium recoil-ion for separate scattering processes. Taking into account the law of pulse energy conservation, it gave an opportunity to fully restore the kinematic properties of the scattering process. Moreover, the use of the method solved the problem of a very low cross-section of the Compton ionization in the photon energy diapason of several KeV that is about 6 times lower than the typical cross-section of the photoabsorption. All this opens opportunities for the use of Compton scattering as yet another instrument of atomic spectroscopy along with such powerful methods of study of atoms and molecules as (e, 2e), (ion, ion e) and others. Theoretical description of this phenomenon is based on the calculations carried out at the supercomputer “Govorun”.
Following information by LIT
We also offer to your attention the article “New Opportunities of the Compton Effect” by O. Chuluunbaatar, Yu. V. Popov and I. P. Volobuev published in the 3rd issue of the bulletin “JINR News” 2020.
An international research group, in which the authors of this article participated within international cooperation of JINR, Moscow State University and Goethe University (Frankfurt am Main, Germany), carried out an experimental measurement of the characteristics of the Compton scattering at free helium atoms using the COLTRIMS (COLd Target Recoil Ion Momentum Spectroscopy) detector at the Petra III synchrotron (DESY, Hamburg) and provided a relevant theoretical description of the obtained results. Compton scattering of photons with an energy of 2.1 keV by helium atoms near the ionization threshold, i.e., the reactions in which the transferred energy is close to the potential of single ionization of the helium atom Ip = 24.6 eV, was observed in the experiment [1]. As a result, there was found a noticeable difference between the observed angular distribution of radiation scattered by bound electrons and the angular distribution of radiation scattered by free electrons, which is given by the Thomson formula.
Almost 100 years ago, in 1922–1923, the American physicist Arthur Compton studied the phenomenon of a change in the wavelength of light scattered on graphite with the emission of an electron. He discovered that the value of the shift of the scattered spectrum to the region of large wavelengths increased with the increasing scattering angle. A theoretical description of the Compton scattering at free electrons was provided by Compton himself in the 1920s [2] on the basis of the concept of a photon as a relativistic particle. This description was also independently found by P. Debye [3]. As is known, at that time there was a famous discussion among physicists about the nature of light, and the discovery of the Compton effect became one of the most convincing arguments in favour of the corpuscular theory. For this discovery, Compton won the Nobel Prize in physics in 1927.
Shortly after the development of quantum mechanics and the description in its framework of the structure of atoms, J. DuMond [4] made the assumption based on the results of his experiments that using Compton scattering it was possible to judge the structure of atoms of the scatterer. He related the broadening of the energy spectrum observed at a fixed scattering angle with the momentum distribution of bound electrons in the scattering matter, supposing that the shifted line broadened as a result of scattering of radiation at randomly moving electrons of the scatterer, similar to the way the Doppler broadening of optical lines of moving atom radiation occurs. Having examined several test momentum distributions for different electron states, DuMond discovered that the structure of the observed spectrum of radiation scattered at beryllium atoms was well reproduced theoretically if the quantum-mechanical description of bound electrons in atoms was used.
Since Compton’s experiments, experiments in this field were based on the coincidence method for simultaneous detection of an electron and a scattered photon emitted by ionization, which was proposed by the German physicist W.Bothe to study the Compton effect in 1924. In 1954, Bothe was awarded the Nobel Prize “for the coincidence method and his discoveries made therewith” [5]
However, the use of the electron–photon coincidence method for precision measurements is impossible due to a number of technical restrictions. The situation changed after the invention of a new method for detecting scattered particles, which was named COLd Target Recoil Ion Momentum Spectroscopy (COLTRIMS) [6], and a real opportunity to use the Compton scattering for determining angular and energy spectra of both scattered photons and electrons emitted by ionization appeared. The COLTRIMS method allows one to simultaneously measure the momenta of an electron and a recoil ion, which makes it possible to carry out measurements by the coincidence method with high accuracy. In particular, using this method, it became possible to collect ions and electrons from almost the full solid angle Ωfull = 4π. In this case, the momentum of a scattered photon can be found from the law of conservation of momentum, as a result of which there is no need to detect the photon itself
In quantum electrodynamics, the standard theory of Compton ionization is based on two Feynman diagrams (Fig.1), however, in the case of photons with an energy of several keV, it makes sense to consider the description of this process using the non-relativistic Schrödinger equation [7]. As a result, the matrix element corresponding to these diagrams splits into the sum of two terms. Both of these terms are of the second order in electron charge; however, the first term traditionally denoted by A2 resembles in form the first Born approximation in the case of atom ionization by a charged particle (proton, electron), and the second (integral) term coincides in form with the second Born approximation. At the selected photon energy, the term A2 makes the major contribution, while the second term is extremely small and plays the role of correction.
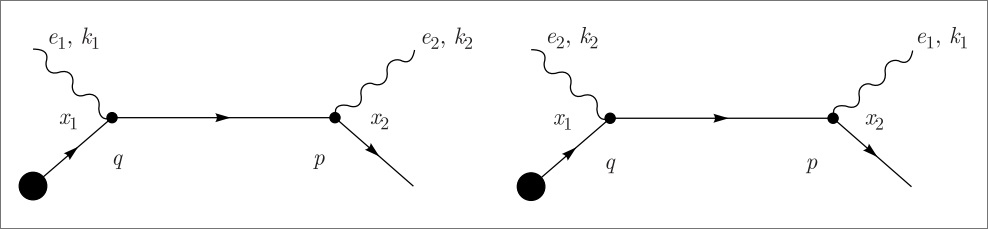 Fig.1. Feynman diagrams for the Compton scattering
Fig.1. Feynman diagrams for the Compton scattering
This theoretical model turned out to be quite simple, which allowed one to consider a number of test functions of the initial and finite states and compare the results with the experiment, as well as to evaluate the possibility of this new method to carry out precision spectroscopy (angular and energy) of the outermost shells of an atom (molecule). The calculations were performed at the “Govorun” supercomputer of JINR, and the obtained results were in a good agreement with the experiment (Fig.2). At the same time, the experiments distinguished sets of test functions, which showed the possibility of using Compton ionization along with the well-known spectroscopic methods, such as (е, 2е), (р, ре), etc.
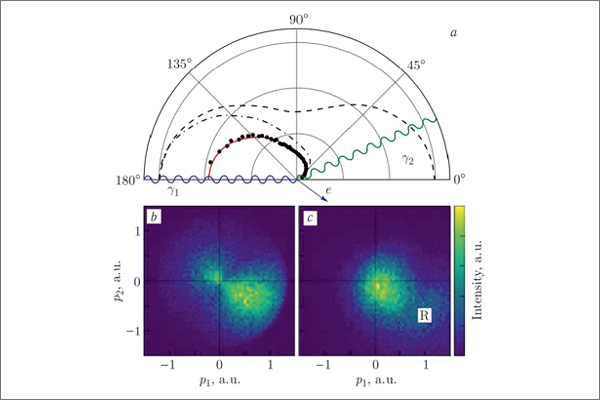 Fig. 2. Scheme of ionization by the Compton scattering at ω = 2.1 keV. a) The wavy lines indicate the incoming and scattered photons, and the arrow depicts the momentum vector of the emitted electron. The dashed line shows the Thomson cross section, i.e., the angular distribution of a photon scattering at a free electron. The black dots show the experimental photon angular distribution for ionization of He by the Compton scattering, integrated over all electron emission angles and energies below 25 eV. The statistical error is smaller than the dot size. The black dash-dotted line shows the A2 approximation for all electron energies, and the solid red line shows the A2 approximation for electron energies below 25 eV. The black dashed and dash-dotted lines are multiplied by a factor of 1.9. b) Momentum distribution of electrons emitted by the Compton scattering of 2.1 keV photons at He. The coordinate frame is the same as in a, i.e., the plane is defined by the incoming (horizontal) and scattered photon (upper half plane). The momentum transfer points to the forward lower half plane. The data are integrated over the out-of-plane electron momentum components. c) He+ ion momentum distribution for the same conditions as in b
Fig. 2. Scheme of ionization by the Compton scattering at ω = 2.1 keV. a) The wavy lines indicate the incoming and scattered photons, and the arrow depicts the momentum vector of the emitted electron. The dashed line shows the Thomson cross section, i.e., the angular distribution of a photon scattering at a free electron. The black dots show the experimental photon angular distribution for ionization of He by the Compton scattering, integrated over all electron emission angles and energies below 25 eV. The statistical error is smaller than the dot size. The black dash-dotted line shows the A2 approximation for all electron energies, and the solid red line shows the A2 approximation for electron energies below 25 eV. The black dashed and dash-dotted lines are multiplied by a factor of 1.9. b) Momentum distribution of electrons emitted by the Compton scattering of 2.1 keV photons at He. The coordinate frame is the same as in a, i.e., the plane is defined by the incoming (horizontal) and scattered photon (upper half plane). The momentum transfer points to the forward lower half plane. The data are integrated over the out-of-plane electron momentum components. c) He+ ion momentum distribution for the same conditions as in b
Thus, the experimental and theoretical results recently published in Nature Physics [1] showed new opportunities of the Compton ionization of an atom with the energy transfer close to the threshold of single ionization as an effective method of spectroscopy of the outermost shells of atoms and molecules. This became possible due to the precision measurements of very small differential cross sections using modern technical tools and theoretical calculations of these cross sections at the “Govorun” supercomputer of JINR. As a result, pioneers’ attempts to use the Compton effect, which was discovered almost 100 years ago, for spectroscopy of quantum objects using then imperfect technologies have gained a new impetus today.
References
- Kircher M. et al. Kinematically Complete Experimental Study of Compton Scattering at Helium Atoms near the Threshold // Nature Phys. 2020. V. 16. P.756–760.
- Compton A.H. // Phys. Rev. 1923. V.21. P.483.
- Debye P. // Z. Physik. 1923. V. 24. P. 161.
- DuMond J.W.M. // Phys. Rev. 1929. V.33. P.643.
- Bothe W. Nobel Lecture. 1954.
- Ullrich J. et al. // Rep. Prog. Phys. 2003. V.66. P.1463.
- Bergstrom P.M., Jr., Surić T., Pisk K., Pratt R.H. // Phys. Rev. A. 1993. V.48. P.1134.